By: Tao Steven Zheng (郑涛)

【Problem】

Begin with the relativistic momentum and energy:

Derive the relativistic energy-momentum relation:

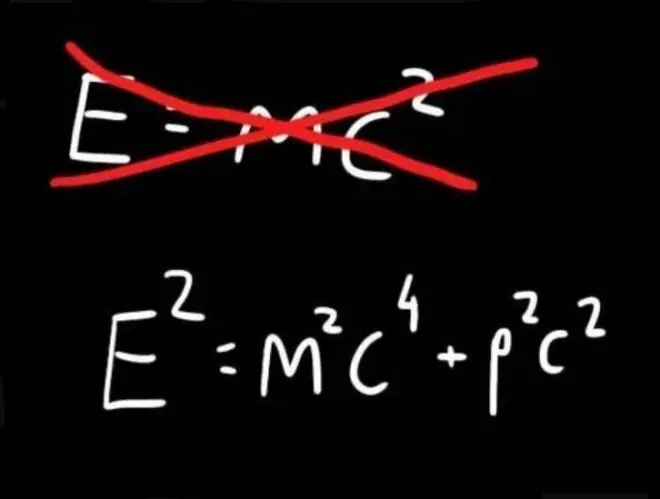
【Solution】

With a little algebra we discover that

Square the equation for relativistic energy

And rearrange to arrive at

From the relation

we find

and

Substitute this result into

to get

Note: When the momentum of an object is zero, the expression reduces to Einstien's famous mass-energy equivalence principle .